Waves

Properties, polarisation, stationary waves

Waves

Types of Waves

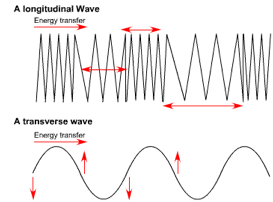
·      Longitudinal waves are caused by a vibration that is parallel to the direction of the wave e.g. sound

·      Transverse waves are caused by a vibration that is perpendicular to the direction of the wave e.g. electromagnetic waves, waves on a string

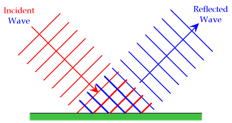
The energy is passed through the wave even though individual particles only vibrate The difference between transverse and longitudinal waves is the direction the particles vibrate compared to the direction of the energy transfer A compression is where the particles bunch together A rarefaction is where they are spread out http://lgfl.skoool.co.uk/uploadedImages/long_trans_wave.gif

 

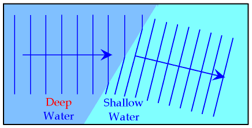
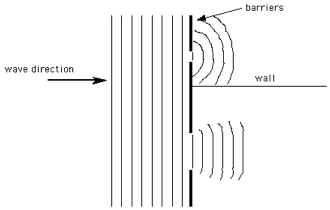
Properties of Waves

c = f _The wave equationNotice that there is distance on the x-axis which makes it possible to measure the wavelength in metres. Sometimes the x-axis is time (s) instead e.g. on an oscilloscope trace. In this case the ‘length’ of one complete wave would actually represent the time period, T of the wave http://seagrant.uaf.edu/marine-ed/curriculum/images/stories/grade7/wave.jpg

 

 


f = 1/Tc= wave speed (or velocity)

f = frequency (Hz) – how many waves pass a point per second

_ = wavelength (m)

T = time period – time for one complete wave to pass a point (s)

Phase

radians=(degrees x π)/180If two waves are ‘in step’ with each other we say they are ‘in phase’ or have a phase difference of 0. For all other waves there is a difference in phase between them. 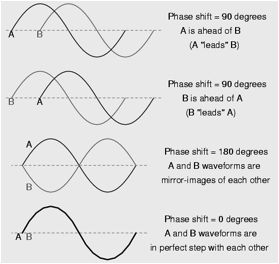If they are completely ‘out of step’ we say they are ‘out of phase’ or have a phase difference of 1800 Any phase angle is possible Phase is normally measured in degrees or radians: 3600 = 2π radians 1800 = π radians 900 = π/2 radians http://sub.allaboutcircuits.com/images/02021.png

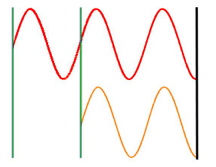
http://www.visionlearning.com/library/modules/mid132/Image/VLObject-3401-050617100618.jpg

·      Sometimes we also use the term ‘path difference’ to describe the difference between two waves

for example – the red wave here has travelled a distance of 1 wavelength or _ further than the orange wave – but they are still in phase (phase difference of 0 or 3600

·      A path difference of _/2 would make 2 identical waves 180 out of phase

 

 

http://www.cyberphysics.co.uk/graphics/diagrams/light/polarisation2.png

Polarisation

·      Only transverse waves have polarisation

·      It is a measure of the angle of vibration as viewed along the axis of the wave

·      Unpolarised waves have all angles present

·      A polaroid is a material that can polarise light in one angle

 

 

 

Wave Fronts

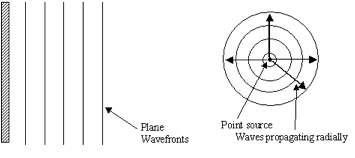http://www.antonine-education.co.uk/Image_library/Physics_2/Waves/Wave_properties/wav_2.gifWaves produced by a ripple tank are called wave fronts

 

Each line represents the crest of a wave

 

The distance between two wave fronts in the wavelength

 

RefractionReflection http://www.smkbud4.edu.my/Data/sites/vschool/phy/wave/Refraction-Water-Waves.gif

 

The narrower the gap the more the diffractionDiffractionhttp://www.district196.org/schools/avhsold/dept/science/physics/physicsweb04/AVHSPhysics/images/diffraction%20pic.GIF

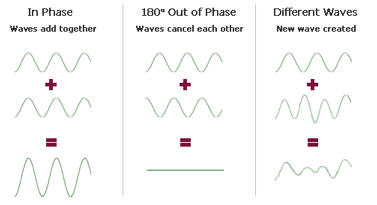
Superposition

·      The principle of superposition is that when two waves meet, the total displacement at a point is equal to the sum of the individual displacements at that point

 

 

 

http://www.mediacollege.com/audio/images/wave-interaction.gif

Stationary Waves

·      A wave that moves through a medium and carries energy from one place to another is called a progressive wave

·      A stationary wave (sometimes referred to as a ‘standing wave’)

-   Does not transfer energy (it stores it)

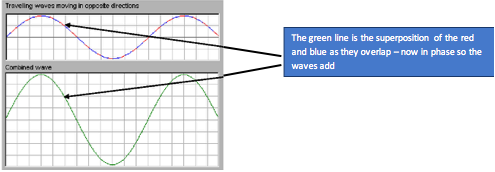
-   Is formed when two progressive waves pass through each other in opposite directions

-   When the waves have the same frequency/wavelength and similar amplitude

-   Can be formed in many different places e.g. on a vibrating string, by sound in a tube, by microwaves reflecting from metal plates

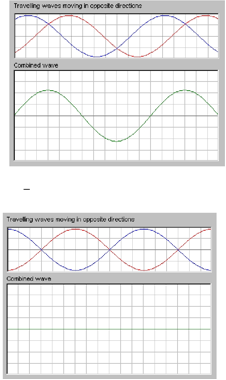
-   Only occur when the progressive waves have certain frequencies

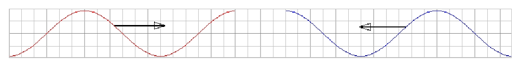
Imagine two progressive waves of equal wavelength/frequency and the same amplitude meeting from opposite directions.:

Time Displacement The time period of the wave is 12 squares
The red wave is moving right and the blue wave left – now the time has advanced by T/8 from the previous diagram i.e. the red wave has moved 1.5 squares to the right and the blue wave 1.5 squares to the left. The new superposition is shown – it has a lower amplitude
Now the time has advanced to T/4. Each wave has advanced a total of 3 squares. They are now in anti-phase and so the superposition is level
This process continues as the progressive waves continually move through each other. The net effect is that each point on the combined waves oscillates up and down and a stationary wave is formed

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 


 


BA• Stationary waves are often draw like this • The red and blue lines here are the wave envelopes – the string is oscillating up and down between those limits • You need to be able to label nodes and anti-nodes on these diagrams • Points on stationary waves separated by a node are 1800 out of phase e.g. A and B http://cnx.org/content/m12413/latest/StandingWaveHarmonics.png

Node Anti-node
 

 


Using the wave equation and the wavelength of the wave compared to the length of the gap gives formula for the frequency of waves which will produce stationary waves: